CALORIMETRY
Internal energy is measured in terms of work and heat,
This equation is often applied to the first law of thermodynamics. This law states that the energy of the universe remains constant, or energy can be neither created nor destroyed, only changed from one form of energy to another. The sign of dq, and dw, indicates the direction of the flow of energy (+ means in, - means out). We define dwexp as the work done by a system expanding against an external pressure,
and dwother is non expansion work (such as electrical work). It becomes clear that the change in internal energy of a system is not equal to the amount of heat supplied at constant pressure if the system is free to change its volume. This quantity is equal to the change in the enthalpy of the system,
for a system that can only do expansion work,
and since we know that dwexp = -P dV, we obtain for the enthalpy change:
if the reaction occurs at constant pressure, as it does if the reaction vessel is open to the atmosphere, then dp = 0
or ΔH = qp for measurable changes. This means that when a system is subjected to constant pressure and only expansion work is possible, the change in enthalpy is equal to the thermal energy input/output.
An enthalpy change can be measured using an isobaric calorimeter. ΔH and ΔU differ by an amount pΔV. So if we know the enthalpy change, the volume change, and the external pressure of the system, we can compute the internal energy change. For example:
Certain compound converts to an alternate allotropic form
where the subscripts indicate the molar volume of each allotropic form in g/cm3. The internal energy change of this process is -0.25 kJ/mol at 1.0 atm of pressure. Compute the percent difference between the enthalpy change and the internal energy change for the process.
1.0 atm = 101325 Pa
pΔV = 101325 Pa X (27 - 31) X 10-6 m3 = -0.4053 X 10-3 kJ
% difference = [-0.4053 X 10-3 kJ / -0.25 kJ/mol] = 0.16%
Thus it is reasonable to ignore the pΔV contribution for condensed phases. This is not so for reactions that consume or produce gasses. From the ideal gas law we obtain pV = nRT or pΔV = ΔngasRT, and the enthalpy equation becomes:
Going back to the original form of the internal energy equation,
It is clear that if
The internal energy change can also be measured as the change in a single property of the system,
Such measurements are carried out using a bomb calorimeter.
BOMB CALORIMETRY
In this experiment the energy released by the reaction of a limiting reagent. For the change in internal energy of such a system:
Because the reaction takes place in a steel bomb the system cannot change its volume so no work is done. The reaction of choice is the combustion of an organic compound such as naphthalene. In a combustion reaction, the compound (limiting reagent) combines with oxygen (excess reagent) to form CO2 and H2O.
The reaction vessel (the bomb) and its contents are submerged in a pail with a known amount of water, which is placed in a thermally insulated calorimeter. This insulation ensures that the processes in the system are nearly adiabatic. Thus the energy released in the chemical reaction must be transferred entirely as heat (qv) into the system.
Suppose a sample is allowed to come to equilibrium at temperature T0. Upon ignition, the heat released raises the temperature of the system to T1,
where C is the heat capacity of the bomb calorimeter as determined from the calibration step,
For a perfectly adiabatic process we would get the following plot:

And the above equation would be correct. However, no thermal insulation is perfect, some heat will leak in or out of the system during the change of state and the equilibration time. The plot is likely to deviate from the expected adiabatic pattern,
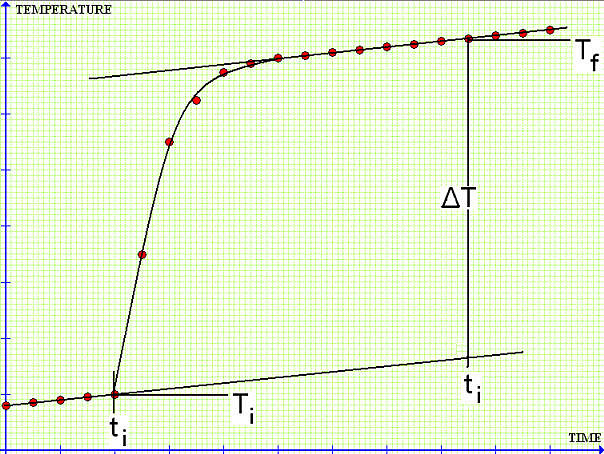
Then, we would estimate ΔT from the tangent to the T-t curves at ti and tf,
Note that the tangent lines are parallel, thus ΔT could be obtained from anywhere in the plot. What if the tangent lines are not parallel?

Determining ΔT. According to the National Bureau of Standards, td is very close to the time at which the temperature has increased by 60% of the difference between ti and tf
Here, ΔT is given by,
In words: if the two tangent lines are parallel then we just have to measure the temperature difference between them; if the two tangent lines are not parallel then we need to take the difference at a certain point td such that the shaded regions are of equal area.
The heat capacity C, has been defined as a single entity. This quantity applies to the bomb calorimeter as long as the amount of water in the pail remains constant. If the amount of water is changed, then we need to redefine the heat capacity as Csys
where Cwater is the heat capacity of the water and Ccal is the heat capacity of the empty calorimeter. For a given amount of water, the heat capacity is:
Thus the heat capacity of the empty calorimeter is,
REQUIRED MATERIALS AND APPARATUS
-Oxygen cylinder and high-pressure fittings and hose connector to the bomb
-Pelletizing press
-Analytical balance
-Benzoic acid tablets for standardization (if tablets are not available, make your own in the pelletizing press)
-Naphthalene and an unknown sample
-Volumetric flasks: 1000.00 mL, 500.00 mL, 100.00 mL
-Adiabatic or plain Bomb calorimeter; this generally consists of the following parts:
*The high-pressure "bomb" made of steel
*The pail, with a known amount of water
*The sample pan
*Ignition wire
*A secondary water jacket to ensure adiabatic conditions for the reaction
*An ignition unit
*A thermometer or automated temperature recording device
*Motor and stirrer
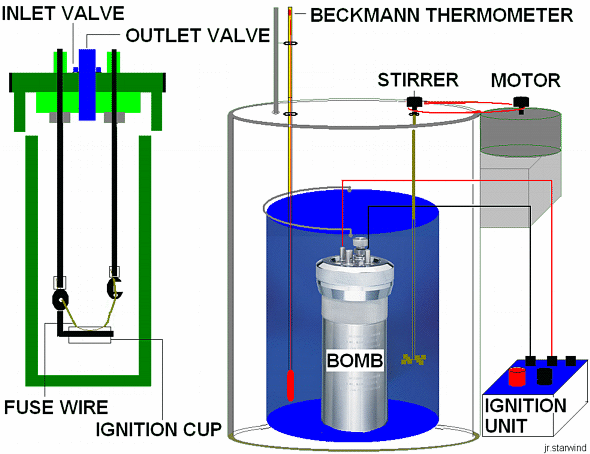 ,
,The bomb calorimeter.
EXPERIMENTAL PROCEDURE
The first step of the experiment is to determine the value of C, the heat capacity of the calorimeter. We do this by measuring ΔT for a known ΔU. We will use high purity benzoic acid, C6H5COOH. The following reaction will occur upon ignition of the sample:
We will determine the heat capacity of the calorimeter from the adiabatic ΔT obtained from the combustion of a known amount of benzoic acid and a known mass of iron wire. The energies of combustion are:
Iron = -6.68 kJ/g
When using powdered samples in a bomb calorimeter, the sample must be compressed into tightly-packed pellets so that the entire sample will combust. The weigh of each sample should not exceed the recommended amount, (~0.6 g for naphthalene and ~0.8 g for benzoic acid) because the bombs are rated to withstand a limited temperature rise.
Never put the calorimeter lid or bomb parts down on the bench top. Use holders supplied to prevent unnecessary damage and have a classmate hold the calorimeter lid while the pail is being replaced/removed.
If the thermometer has been calibrated for total immersion and you want to get fuzzy with the experimental data, correct the thermometer readings for the part that is not immersed in the bath. To do that, place a second thermometer near the exposed stem, the stem correction is given by,
FILLING THE BOMB
-Cut the iron wire, to the correct length (~10 cm) and weight it in an analytical balance. Weigh the recommended amount of sample, record exact mass. Put the solid pellet in the ignition cup and wrap the wire around the ends of the electrode terminals. Bend the wire so that it is in good contact with the pellet but does not touch the ignition cup anywhere. The wire should touch only the terminals and the pellet.
-Taking care to keep the wire connected to the electrodes and in contact with the sample, place the head of the bomb into its container and seal as tightly as possible by hand, ensure that the oxygen outlet valve is open during this time.
-To get the air out of the bomb: First, close the outlet valve on the bomb's top. With the valves on the oxygen tank closed, attach the inlet tube from the tank to the bomb. Close the oxygen valve tightly and charge the bomb with oxygen to ~5-10 atm.
How does the filling process work? If the bomb outlet is closed, the inlet is connected to the oxygen tank, and the release valve in the hose connector is closed, then both the bomb and the hose connector are filled at the pressure shown in the tank's pressure gauge. When the gauge marks the required pressure, close the tank's valve. Now the bomb and the hose connector are filled at the same pressure. If you open the release valve in the hose connector, then the pressure drops to atmospheric pressure. But the bomb's inlet valve only allows gas passage in and not out, then the valve is closed by the excess pressure.
-Now you should bleed the gas out of the bomb by opening the outlet valve on the bomb head until you no longer hear a hissing sound. The bomb is now filled with oxygen at atmospheric pressure and free of air and contaminants. The fill-and empty cycle should be repeated just to make sure that no gasses other than oxygen remain in the bomb. Then fill the bomb to ~25-30 atm (not higher than 30 atm) and disconnect it from the tank.
-Fill the calorimeter's pail with 1500.00-2000.0 mL DI water, the water must be enough to cover the bomb. Place the pail into the insulating jacket and be sure that it sits flat. In order to ensure that the pail is placed correctly, the insulating jacket has a set of bumps which fit into a set of depressions in the bottom of the pail.
-Lower the bomb into the pail, there is a circular depression where the bomb fits. Attach the fuse leads to the connectors on the top of the bomb. Check for gas leaks; if there are any bubbles of gas escaping from around the oxygen bomb DO NOT FIRE THE BOMB.
-Cover the water bath with its lid, connect the stirrer to the motor using the rubber O-ring and insert the temperature probe in the hole. The standard temperature probe is usually a Beckmann thermometer. These thermometers are very fragile and very expensive. BE CAREFUL!
-The Beckmann thermometer has a very narrow reading range (~5-8 degrees), the temperature of the water in the pail should be higher than the lower limit of the calibration range but not too high as to let the heat of reaction take the readings above the upper calibration range. The water temperature might need to be adjusted before filling the pail.
-Start the stirrer and start collecting data, reading the thermometer every 15 sec. It is recommended that you take and record temperature readings for about two minutes before to firing the charge. They will be required to correct ΔT for non-adiabatic conditions. Once the readings are fairly stable, fire the bomb.
-To fire the bomb, hold the ignition button down for 2 to 5 seconds. Do Not hold it down more than 5 seconds. The red light should turn on briefly as you push the button.
-Record the temperature until the readings are fairly stable. Continue to take readings for two minutes after the temperature has reached an apparent state of equilibrium.
-When the run is complete, turn off the stirring motor, lift and move the cover. Lift the pail out of the calorimeter. Remove the bomb and open the gas outlet valve to relieve the inside pressure. Once the pressure has decreased, remove the top of the bomb. When releasing oxygen outlet valve do so under a working fume hood.
-Clean and wipe dry all bomb parts.
-Determine the mass of unburned iron wire and subtract from the initial mass of iron wire.
-If the inside of the bomb is coated with soot, then the oxygen pressure was not large enough. Discard this run and try a higher oxygen pressure but do not exceed 30 atm.
-Repeat the procedure with a naphthalene pellet. Then with an unknown.
DATA PROCESSING
-Plot temperature as a function of time for each run and determine the adiabatic ΔT and the heat capacity C of the calorimeter.
-Determine the ΔU of combustion per gram of weighted samples of naphthalene and the unknown using the adiabatic ΔT and the calorimeter heat capacity.
-Determine the molar ΔU of combustion for naphthalene.
-Since the standard enthalpy and energy of a real gas are defined as the standard enthalpy and energy of the gas in the zero-pressure limit, we can compute the molar enthalpy change ΔHmolar using
-Submit all graphs with your report.


